Question

The sun of the first 3 terms of an arithmetic sequence is 21 while their product is 315 determine these three terms?

Answer 1

The terms of the sequence are x=5 and a=2:

`x + (x + a) + (x + 2a) = 21` →
`5 + (5 + 2) + (5 +2*2) = 21`

`x*(x + a)*(x + 2a) = 315` →
`5*(5 + 2)*(5 + 2*2) = 315`

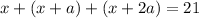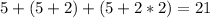
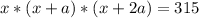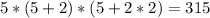
Explanation:

We can find the terms of the following sequence:

`x + (x + a) + (x + 2a) = 21`

`3x + 3a = 21`

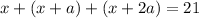
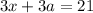
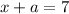`x + a = 7` (1)

The product of that sequence is:

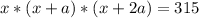`x*(x + a)*(x + 2a) = 315` (2)

Solving equation (1) for x:

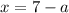`x = 7 - a` (3)

And by entering (3) into (2):

`(7 - a)*(7 - a + a)*(7 - a + 2a) = 315`

`7*(7^(2) - a^(2)) = 315`

`343 - 7a^(2) = 315`

`a = 2`

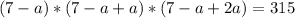
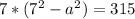
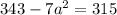
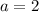
Now, by entering "a" into equation (3):

`x = 7 - 2 = 5`

Therefore, the terms of the sequence are x=5 and a=2.

I hope it helps you!