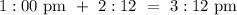Answer:
They will both blink again at 3:12 pm.
Explanation:
As indicated by the question, the way to solve is to find the Lowest (or Least) Common Multiple of both numbers.
As such:

Now knowing the LCM is 132, we convert those minutes into hours and minutes:
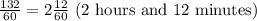
Add this to the original time: