Answer:

Explanation:
To solve by substitution we must first isolate 1 variable in an equation.
Let's use the first equation.

Subtract 3y from both sides to isolate the variable x.
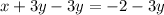

Now we can substitute the expression (-2-3y) in for x in the second equation.
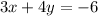
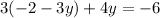
Distribute the 3. Multiply each term inside the parentheses by 3.
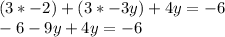
Combine the like terms on the left side.
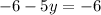
Add 6 to both sides to isolate the variable.

Divide both sides by -5.
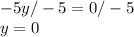
Now we know that y is equal to 0 and can substitute it into the first equation and find x.
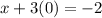
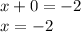
x is equal to -2 and y is equal to 0.