Question

The 100 United States Senators in the 114th Congress, January 2015, included 80 men and 20 women. Suppose 26 of the men and 15 of the women have expressed interested in joining a subcommittee to work on legislation about wage discrimination by gender. In how many ways could a 6 person committee be selected to contain equal numbers of men and women.

Answer 1

The committee can be selected in 1,183,000 ways to contain equal numbers of men and women.

Explanation:

The order in which the people are chosen is not important, which means that the combinations formula is used to solve this question.

Combinations formula:

`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

In how many ways could a 6 person committee be selected to contain equal numbers of men and women.

3 men from a set of 26

3 women from a set of 15. So

`T = C_(26,3)*C_(15,3) = (26!)/(3!23!)*(15!)/(3!12!) = 2600*455 = 1183000`

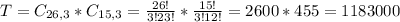
The committee can be selected in 1,183,000 ways to contain equal numbers of men and women.