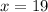Question

Solve x2 + 18x – 19 = 0 by completing the square.

Answer 1

`x = 1`

`x = 19`

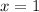
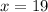
Explanation:

Set up equation

`{x}^(2) + 18x - 19 = 0`

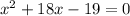
Add 19 on both sides

`{x}^(2) + 18x = 19`

Divide 18 by 2 and sqr it.

`( (18)/(2)) {}^(2)`

`{9}^(2) = 81`

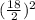
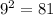
Add 81 on both sides

`{x}^(2) + 18x + 81 = 100`

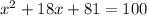
Using the AC method, isolate the left side into a binomial.

`(x + a)(x + b)`

that a and b can be added to equal 18 and be multiplied to equal 81.

Only number that does that is 9. 9+9=18, 9×9=81

`(x + 9)(x + 9)`

`(x + 9) {}^(2)`

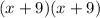
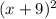
Set up equation

`(x + 9) {}^(2) = 100`

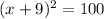
Take sqr root of both sides

`x + 9 = 10`

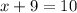
Since sqr root of a positve number can be negative, set up this equation also

`x - 9 = 10`

`x = 1`

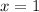
OR

`x = 19`

Answer 2

x = 1, x = -19

Explanation:

1) factorise

(x−1)(x+19)=0

2) equal to 0

x−1=0 or x+19=0

x=1 or x=−19