Question

A player shoots a basketball from a height of 6 feet. The equation, h = -16t^2 + 25t + 6, gives the height, h , of the basketball after t seconds. Describe the height, rounded to the nearest tenth of a foot, of the basketball after 1.5 seconds, assuming no other player touches the ball.

Answer 1

`\boxed {\boxed {\sf 7.5 \ feet}}`

Explanation:

We are given this function for the height (h) after t seconds:

`h=-16t^2+25t+6`

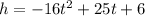
Seconds is t and we want to find the height after 1.5 seconds. Plug 1.5 in for t.

`h= -16(1.5)^2+25(1.5)+6`

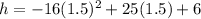
Solve according to PEMDAS: Parentheses, Exponents, Multiplication, Division, Addition, Subtraction.

Solve the exponent first.

• (1.5)²= 1.5*1.5=2.25

`h= -16(2.25)+25(1.5)+6`

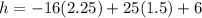
Multiply.

`h= -36+25(1.5)+6`

`h= -36+37.5+6`

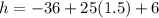
Add.

`h=1.5+6=7.5`

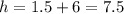
This is already rounded to the nearest tenth, so it is the answer.

After 1.5 seconds, the basketball is at a height of 7.5 feet.