Question

The 1st 3 terms of an A.P., a, a+d, and a+2d, are the same as the 1st 3 terms of a G.P.( a is not equal to 0). Show that this is only possible if r=1 and d=0

Answer 1

See Below.

Explanation:

The first three terms of an A.P is equivalent to the first three terms of a G.P.

We want to show that this is only possible if r = 1 and d = 0.

If a is the initial term and d is the common difference, the A.P. will be:

`a, a+d, \text{ and } a+2d`

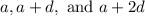
Likewise, for the G.P., if a is the initial term (and it does not equal 0) and r is the common ratio, then our sequence is:

`a, ar,\text{ and } ar^2`

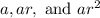
The second and third terms must be equivalent. Thus:

`a+d=ar\text{ and } a+2d=ar^2`

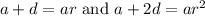
We can cancel the d. Multiply the first equation by -2:

`-2a-2d=-2ar`

We can now add this to the second equation:

`(a+2d)+(-2a-2d)=(ar^2)+(-2ar)`

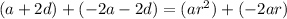
Simplify:

`-a=ar^2-2ar`

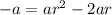
Now, we can divide both sides by a (we can do this since a is not 0):

`-1=r^2-2r`

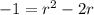
So:

`r^2-2r+1=0`

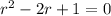
Factor:

`(r-1)^2=0`

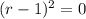
Thus:

`r=1`

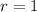
The first equation tells us that:

`a+d=ar`

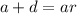
Therefore:

`a+d=a(1)\Rightarrow a+d=a`

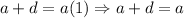
Hence:

`d=0`

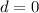
Q.E.D.