Question

Find all the roots for this polynomial using the steps below.

P(X)=x^3+3x^2+3x+2

List the 4 possible rational roots.


Plug each possible rational root into P(x) to determine which is a root of the polynomial.

Divide P(x) by the root you found in part 2 using synthetic division.


Write the fully factored form of P(x).


Use the quadratic formula to find the solutions of the quadratic factor.

List the 3 solutions of the P(x).

Answer 1

`p(x)=(x+2)(x^2+x+1)`

`x=-2`

`x=(-1 + √(3)\:i)/(2)`

`x=(-1- √(3)\:i)/(2)`

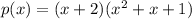
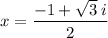
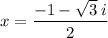
Explanation:

Given polynomial:

`p(x)=x^3+3x^2+3x+2`

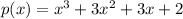
Rational Root Theorem

If P(x) is a polynomial with integer coefficients and if p/q is a root of P(x), then p is a factor of the constant term of P(x) and q is a factor of the leading coefficient of P(x).

Possible p-values

Factors of the constant term: ±1, ±2

Possible q-values

Factors of the leading coefficient: ±1

Therefore, all the possible values of p/q:

`\sf (p)/(q)=(\pm 1)/(\pm 1), (\pm 2)/(\pm 1)=\pm1,\pm2`

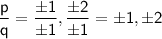
Substitute each possible rational root into the function:

`x=-1 \implies p(-1) =(-1)^3+3(-1)^2+3(-1)+2=1`

`x=1 \implies p(1) =(1)^3+3(1)^2+3(1)+2=9`

`x=-2 \implies p(-2) =(-2)^3+3(-2)^2+3(-2)+2=0`

`x=2 \implies p(2) =(2)^3+3(2)^2+3(2)+2=28`

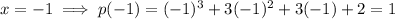
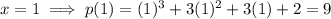
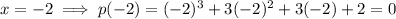
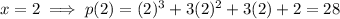
Therefore, x = -2 is a root of the polynomial since f(-2) = 0.

Divide the polynomial by the root using synthetic division:

`\begin{array}c-2 & 1 & 3 & 3 & 2\\\cline{1-1} & \downarrow &-2&-2&-2\\ \cline{2-5} & 1&1&1&0\end{array}`

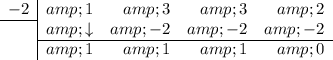
The bottom row (except the last number) gives the coefficients of the quotient. Therefore, the quotient is:

`x^2+x+1`

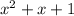
So the fully factored form of p(x) is:

`p(x)= (x+2)(x^2+x+1)`

`\boxed{\begin{minipage}{3.6 cm}\underline{Quadratic Formula}\\\\$x=(-b \pm √(b^2-4ac))/(2a)$\\\\when $ax^2+bx+c=0$ \\\end{minipage}}`

Therefore, the solutions to the quadratic factor are:

`\implies x=(-1 \pm √(1^2-4(1)(1)))/(2(1))`

`\implies x=(-1 \pm √(-3))/(2)`

`\implies x=(-1 \pm √(3\cdot -1))/(2)`

`\implies x=(-1 \pm √(3) √(-1))/(2)`

`\implies x=(-1 \pm √(3)\:i)/(2)`

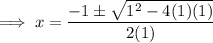
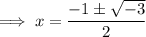
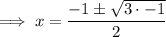
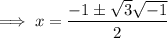
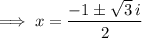
The 3 solutions of p(x) are:

• 
  `x=-2`

• 
  `x=(-1 + √(3)\:i)/(2)`

• 
  `x=(-1- √(3)\:i)/(2)`