Answer:
1) y = -x -1
2) y + 4 = 3( x -2)
3) y = x + 1
Explanation:
1)
The slope is the change in y over the change in x. The y values from the points given is 0 and -1. The x values from the points given is -1 and o
Slope:
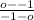 =
=
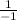 = -1
= -1
y -intercept
y = mx + b Use the point (0,-1) and the slope to solve for b
y = -1
m = -1
x = 0
y = mx + b
-1 = -1(0) + b
-1 = 0 + b
-1 = b This is the y intercept.
y = mx + b
y = =x -1
2)
point-gradient form is
y -y = m (x-x)
y - -4 = 3 (x -2)
y + 4 = 3( x - 2)
3)
Parallel lines have the same slope.
slope is 1. Use this and the point (0,1) to solve for b
y = mx + b
1 = 1(0) + b
1 = 0 + b
1 = b
y = x + 1