Answer:
Yes, the company will break even at some point in time
Explanation:
I am not sure what methodology your teacher has been using. I am going to provide a simple methodology
- The polynomial function for profit is

- If
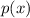 has at least one zero then that means it will intersect the x-axis and the company will break even(Revenue = Costs)
has at least one zero then that means it will intersect the x-axis and the company will break even(Revenue = Costs)
- The zeros of
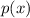 are the values of x where
are the values of x where
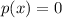
- So if we can find out if
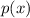 ever crosses the x-axis then that would indicate that the company will break-even at some value of
ever crosses the x-axis then that would indicate that the company will break-even at some value of
 years. If not, the company will never break even.
years. If not, the company will never break even.
- There is a rule to determine how many zeros a polynomial will have or in other words how many times it crosses the x-axis. This is known as Descartes' Rule of Signs.
- Briefly the rule states that the number of zeros can be determined by the number of sign changes in the polynomial for consecutive terms
- p(x) can be re-written as:
+x³ - 4x² - 1
- If we look at the signs we see + - -
- Therefore there is one sign change from + to -
- This means p(x) will have one zero
Therefore the company will break-even at some point
Other Notes
- You can also solve for the roots of the polynomial and see how many real roots are there. In this case there is one real root at
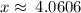 . But that is a lot of work
. But that is a lot of work
- Another way to approach this problem is to simply graph the function and see if it crosses the x axis. I have provided the graph of this function and you can see that it does cross the x-axis at
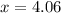
I hope that helps you