Answer:
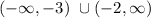
====================================================
Step-by-step explanation:
Let's solve the first inequality.
4(x+1) > -4
4x+4 > -4
4x > -4-4
4x > -8
x > -8/4
x > -2
The inequality sign doesn't flip since we didn't divide both sides by a negative value.
Now let's solve the other inequality.
2x-4 < -10
2x < -10+4
2x < -6
x < -6/2
x < -3
-----------------------------------
To summarize:
- solving 4(x+1) > -4 leads to x > -2
- solving 2x-4 < -10 leads to x < -3
Draw out a number line. Place open holes at -3 and -2.
Shade to the right of -2 and to the left of -3 to indicate x > -2 and x < -3 respectively.
See the diagram below.
The interval in red is x < -3. This is the same as
 and that becomes the interval notation
and that becomes the interval notation
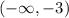
The interval in blue is x > -2. It is the same as
 which becomes the interval notation
which becomes the interval notation
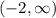
Glue those interval notations together to end up with the final answer of
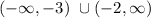
The "U" is the union symbol.