Answer:
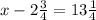
(where x is the original length of the pipe).
Original length of the pipe = 16 feet
Explanation:
Let x be the original length of the pipe.
Given a plumber cuts 2 3/4 feet from a pipe and now has a pipe that is 13 1/4 feet long, the equation that models this is:
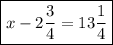
To determine the length of the original pipe, solve the equation for x.
Add 2 3/4 to both sides of the equation:
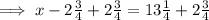
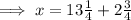
When adding mixed numbers, partition the mixed numbers into fractions and whole numbers, and add them separately:
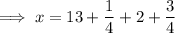
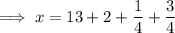
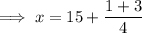
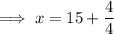
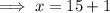
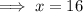
Therefore, the original length of the pipe was 16 feet.