Question

Find the volume of the cone where Radius= 3 and height=7 .
Please use π = 22/7
​

Answer 1

`\displaystyle \boxed{\tt \: VOLUME \; OF\; THE\; CONE = 66 \;units^3 }`

Explanation:

Given:

Radius [r] = 3

Height [h] =7

To Find:

Volume of the cone

Solution:

We need to use the formula of volume of cone to find the volume of cone.

So We know that,

`\boxed{\rm \: Volume \: of \: the \: cone = \tt \cfrac{1}{3} \pi{r} {}^(2) h}`

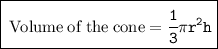
Where,

• π = 22/7[According to the question]
• r = radius
• h = height

So put their values in the formulae:

• r = 3
• h = 7

`\rm \: Volume \: of \: the \: cone = \cfrac{1}{3 } * \cfrac{22}{7} * 3 {}^(2) * 7`

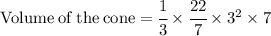
Now Simplify to find the value of cone.

`\rm \: Volume \: of \: the \: cone = \cfrac{1}{3} * \cfrac{22}{7} * 3 * 3 * 7`

`\rm \: Volume \: of \: the \: cone = \cfrac{1}{3} * \cfrac{22}{7} * 9 * 7`

`\rm \: Volume \: of \: the \: cone = \cfrac{1}{3} * \cfrac{22}{7} * 63`

`\rm \: Volume \: of \: the \: cone = \cfrac{1}{3} * \cfrac{22}{ \cancel{7}{}^1} * \cancel{63}\; \; {}^(9)`

`\rm \: Volume \: of \: the \: cone = \cfrac{1}{3} * 22 * 9`

`\rm \: Volume \: of \: the \: cone = \cfrac{1}{3} * 198`

`\rm \: Volume \: of \: the \: cone = \cfrac{1}{ \cancel{3} {}^(1) } * \cancel{{ 198} } \: ^(66)`

`\rm \: Volume \: of \: the \: cone = 1 * 66`

`\boxed{ \rm \: Volume \: of \: the \: cone = \boxed{\rm 66 \: \: units {}^(3)}}`

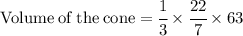
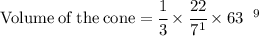
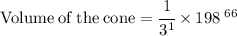
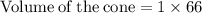
Hence, the volume of the cone would be 66 units^3 .

`\rule{225pt}{2pt}`

I hope this helps!

Answer 2

Given -

♦ Radius of cone = 3 units

♦ Height = 7 units

`volume = (1)/(3) \pi \: r {}^(2) h \\ \\ = > \frac{1}{\cancel{3}} * \frac{22}{\cancel{7} } * \cancel{ 3} * 3 * \cancel{7} \\ \\ = > 22 * 3 \\ \\ = > 66 \: units {}^(3)`

Go for it :D