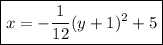Question

The vertex form of the equation of a horizontal parabola is given by x=1/4p(y-k)2+h , where (h, k) is the vertex of the parabola and the absolute value of p is the distance from the vertex to the focus, which is also the distance from the vertex to the directrix. You will use GeoGebra to create a horizontal parabola and write the vertex form of its equation. Open GeoGebra, and complete each step below.

Part A
Mark the focus of the parabola you are going to create at F(-5, 2). Draw a vertical line that is 8 units to the right of the focus. This line will be the directrix of your parabola. What is the equation of the line?

Part B
Construct the line that is perpendicular to the directrix and passes through the focus. This line will be the axis of symmetry of the parabola. What are the coordinates of the point of intersection, A, of the axis of symmetry and the directrix of the parabola?

Part C
Explain how you can locate the vertex, V, of the parabola with the given focus and directrix. Write the coordinates of the vertex.

Part D
Which way will the parabola open? Explain.

Part E
How can you find the value of p? Is the value of p for your parabola positive or negative? Explain.

Part F
What is the value of p for your parabola?

Part G
Based on your responses to parts C and E above, write the equation of the parabola in vertex form. Show your work.

Part H
Construct the parabola using the parabola tool in GeoGebra. Take a screenshot of your work, save it, and insert the image below.

Part I
Once you have constructed the parabola, use GeoGebra to display its equation. In the space below, rearrange the equation of the parabola shown in GeoGebra, and check whether it matches the equation in the vertex form that you wrote in part G. Show your work

Part J
To practice writing the equations of horizontal parabolas, write the equations of these two parabolas in vertex form:

focus at (4, 3), and directrix x = 2
focus at (2, -1), and directrix x = 8

Answer 1

A. x = 3

B. A = (3, 2)

C. V = (-1, 2)

D. Left

E. See below.

F. p = -4

`\textsf{G.} \quad x=-(1)/(16)(y-2)^2-1`

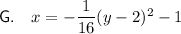
H. See attachment.

`\textsf{J.} \quad x=(1)/(4)(y-3)^2+3`

`x=-(1)/(12)(y+1)^2+5`

Explanation:

Part A

The given focus of the parabola is F (-5, 2).

The equation for a vertical line is x = a.

Therefore, a vertical line that is 8 units to the right of the focus is:

`\implies x=-5+8=3`

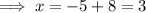
Therefore:

• Directrix: x = 3

Part B

A line that is perpendicular to the directrix is a horizontal line.

The equation for a horizontal line is y = a.

As the horizontal line passes through the focus:

• Axis of symmetry: y = 2

The point of intersection, A, of the axis of symmetry and the directrix is:

• (3, 2)

Part C

The vertex of the parabola is the point that is halfway between the focus and the directrix.

Therefore:

• Vertex = (-1, 2)

Part D

The parabola will open sideways as the axis of symmetry is horizontal.

As a parabola never touches its directrix, this parabola will open to the left.

Part E

The absolute value of p is the distance between the vertex and the focus, and the distance between the vertex and the directrix.

For a sideways parabola, if p > 0 the parabola opens to the right, and if p < 0 the parabola opens to the left.

Part F

Since the focus and directrix are 8 units apart, and the parabola opens to the left:

• p = -4

Part G

Vertex form of a sideways parabola:

`\boxed{x=(1)/(4p)(y-k)^2+h}`

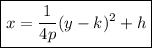
Given:

• Vertex = (-1, 2) ⇒ h = -1, k = 2
• p = -4

Substitute the values into the formula:

`\implies x=-(1)/(16)(y-2)^2-1`

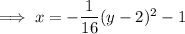
Part H

See attachment.

Part I

Rearrange the equation of the parabola shown in the attachment:

`y^2+16x-4y=-20`

`\implies 16x=-y^2+4y-20`

`\implies 16x=-(y^2-4y+4)-16`

`\implies 16x=-(y-2)^2-16`

`\implies x=-(1)/(16)(y-2)^2-1`

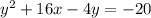
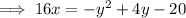
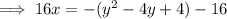
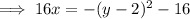
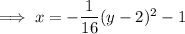
This matches the equation in vertex form in part G.

Part J

Given:

• Focus = (4, 3)
• Directrix: x = 2

Therefore:

• Vertex = ((4+2)/2, 3) = (3, 3)
• Parabola opens to the right, so p > 0.
• p = |4 - 3| = 1

Therefore, the equation of the parabola with the given parameters is:

`\boxed{x=(1)/(4)(y-3)^2+3}`

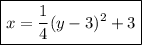
Given:

• Focus = (2, -1)
• Directrix: x = 8

Therefore:

• Vertex = ((8+2)/2, -1) = (5, -1)
• Parabola opens to the left, so p < 0.
• p = -|2 - 5| = -3

Therefore, the equation of the parabola with the given parameters is:

`\boxed{x=-(1)/(12)(y+1)^2+5}`