Question

PLEASE PROVIDE EXPLANATION!

In a two-digit number the units digit is three less than the tens digit. If the digits are reversed, the sum of the reversed number and the original number is 121. Find the original number.

Answer 1

The original number is 74

Explanation:

The answer of 74 provided by brey90 is absolutely correct. I am just providing a mathematical way of determining the number

Let the number be ab
That means
`b` is in the units place and
`a` is in the tens place.

The actual value of this two digit number is
`10a + b`

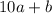
The difference between a and b is 3:

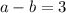`a - b = 3` [1]

If we reverse the number we get the number
`ba`

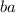
The actual value of
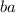`ba` is
`10b + a`

The sum of
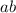`ab` and
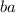`ba` is 121. This means the sum of their actual values must be 121

So we get

`10a + b + 10b + a = 121\\\\11a + 11b = 121\\\\`

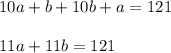
Dividing both sides by 11 yields:

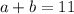`a + b = 11` [2]

Add [1] and [2] to get:

`2a = 14\\\\a = 7\\\\`

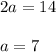
Sub for a in Equation [1] to get

`7 - b = 3\\\\b = 4\\\\`

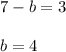
So the original number is 74

Check
Reversed is 47
74 + 47 = 121

Answer 2

Explanation:

Also, we know that , because the unit digit is three less than tens digit, or tens digit is three more units than the unit digit. Then, the sum of the reversed number and the original number is 121, this would be expressed: Therefore, the digits are 7 and 4. The number would be 74.