Question

Graph each function and identify its domain, range, intercepts, asymptotes, and holes.

Thank you! :)

Answer 1

• Domain: real numbers except {-4, -3, 0}
• Range: real numbers except {2}
• Intercepts: (-1, 0); no y-intercept (see Holes)
• Asymptotes: horizontal, y = 2; vertical, x = -3
• Holes: (-4, 6), (0, 2/3)

Explanation:

You want the domain, range, intercepts, asymptotes, and holes of the rational function f(x) = (2x³ +10x² +8x)/(x³ +7x² +12).

Simplified

The given function can be simplified by cancelling common factors from numerator and denominator. These cancelled factors show where the holes are in the graph.

`f(x)=(2x^3+10x^2+8x)/(x^3+7x^2+12)=(2x(x+4)(x+1))/((x(x+4)(x+3))=2\left((x+1)/(x+3)\right)\quad x\\otin\{-4,-3,0\}`

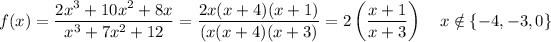
Domain

The function is defined for all real numbers except those where the denominator is zero: {-4, -3, 0}.

Range

The function can produce every output value except the value of the horizontal asymptote: y = 2. The range is all real numbers except 2.

Intercepts

The x-intercept is found where the numerator of the simplified function is zero: x = -1

The y-intercept is the function value at x=0. The function is undefined there, so the y-intercept does not exist. (The limit as x approaches zero is y = 2/3.)

Asymptotes

The function has a horizontal asymptote at the y-value corresponding to the ratio of the highest-degree terms of the numerator and denominator: y = 2.

The vertical asymptote is at the uncanceled denominator zero, x = -3.

Holes

The holes are where a numerator and denominator factor cancel. The y-value at the hole is the limit of the function value as x approaches the hole location. This is the value of the simplified function.

The holes are (-4, 6) and (0, 2/3).