Answer:
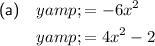

Explanation:
Part (a)
A graph is symmetric with respect to the y-axis if for every point (a, b) on the graph, there is also a point (-a, b) on the graph.
Linear functions are not symmetric with respect to the y-axis.
Quadratic functions are symmetric with respect to the y-axis if the y-axis is their axis of symmetry, so when the variable x is squared.
Cubic functions are not symmetric about the y-axis.
Square root functions are not symmetric about the y-axis.
There are two quadratic functions with x² in the given answer options.
Test to see if they are symmetric with respect to the y-axis.
Test for symmetry
To determine if a graph is symmetric with respect to the y-axis, replace all the x's with (−x). If the resultant expression is equivalent to the original expression, the graph is symmetric with respect to the y-axis.
Given function:
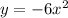
Replace x with (-x):
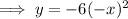

Therefore, as the function is equal to the original function, this function is symmetric with respect to the y-axis.
Given function:
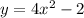
Replace y with (-y) and x with (-x):
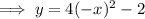

Therefore, as the function is equal to the original function, this function is symmetric with respect to the y-axis.
Part (b)
A graph is symmetric with respect to the x-axis if for every point (a, b) on the graph, there is also a point (a, -b) on the graph.
Linear functions are not symmetric with respect to the x-axis.
Quadratic functions are symmetric with respect to the x-axis if the x-axis is their axis of symmetry, so when the variable y is squared.
Cubic functions are not symmetric about the x-axis.
Square root functions are not symmetric about the x-axis.
There is one quadratic functions with y² in the given answer options.
Test to see if it is symmetric with respect to the x-axis.
Test for symmetry
To determine if a graph is symmetric with respect to the x-axis, replace all the y's with (−y). If the resultant expression is equivalent to the original expression, the graph is symmetric with respect to the x-axis.
Given function:
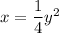
Replace y with (-y):
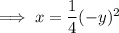
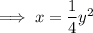
Therefore, as the function is equal to the original function, this function is symmetric with respect to the x-axis.