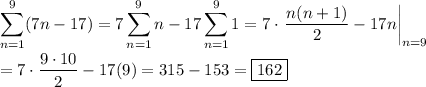Question

\sum _{n=1}^{9}(7n-17)
n=1
∑ (7n−17)
9

Answer 1

162

Explanation:

You want the sum of the first 9 terms described by ...

a[n] = 7n -17

Add the terms

There are several ways the sum can be found. Most straightforward is simply adding up the terms:

For n=1, 7n-17 = -10

For n=2, 7n -17 = -3

The remaining 7 terms are 4, 11, 18, 25, 32, 39, 46.

The sum is ...

-10 -3 +4 +11 +18 +25 +32 +39 +46 = 162

Arithmetic sequence

The terms form an arithmetic sequence with first term -10 and last term 46. The sum will be the average term multiplied by the number of terms:

((-10 +46)/2)(9) = 18(9) = 162

Sum formulas

The sum can be decomposed into the sums whose formulas we know:

`\displaystyle \sum_(n=1)^9(7n-17)=7\sum_(n=1)^9{n}-17\sum_(n=1)^9{1} =7\cdot\left.(n(n+1))/(2)-17n\right|_(n=9)\\\\= 7\cdot(9\cdot10)/(2)-17(9)=315-153=\boxed{162}`