Question

1. Which equation passes through the points (3,-5) and (-1, 3)?

(1) y + 5 = x-3
(2) y = 2x + 1
(3) y-3 = -2(x + 1)
(4) y=x+2

Answer 1

3)y-3=-2(x+1)

Explanation:

y-3=-2(x+1)

add 3 on both sides and use distributive property with the -2

y=-2x-2+3

y=-2x+1

graph it.

Answer 2

(3) y-3 = -2(x + 1)

Explanation:

Lets change all equations to slope-intercept form to easily see the slope.

Slope-Intercept Form:

y = mx+b

M is slope, or the amount the y-value increases when the x-value increases by 1. B is y-intercept, or the y-value of the line when it is intersecting the y-axis (x=0).

#1: y + 5 = x - 3

Subtract 5 on both sides.

y + 5 - 5 = x - 5

Simplify.

y = x - 5

In slope intercept form. Slope of 1 and y-intercept of -5.

#2: y=2x+1

Already in slope intercept form. Slope of 2 and y-intercept of -5.

#3: y-3 = -2(x+1)

Use distributive property to simplify.

y - 3 = -2x-2

Add 3 on both sides.

y - 3 + 3 = -2x - 2 + 3

Simplify.

y = -2x+1

In slope intercept form. Slope of 2 and y-intercept of 1.

#4: y = x+2

Already in slope intercept form. Slope of 1 and y-intercept of 2.

Now using the slope formula, we can eliminate 2 possibilities.

Slope Formula:
`(y_2-y_1)/(x_2-x_1)`

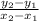
Plug in points.

`(3--5)/(-1-3)`

Simplify.

`(8)/(-4)`

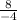
Divide.

-2

Slope is -2. Because the slopes of #1 and #4 are 1 (not -2), they cannot be the equation that passes through the line. The slope of #2 is 2, not -2. We are left with: #3.