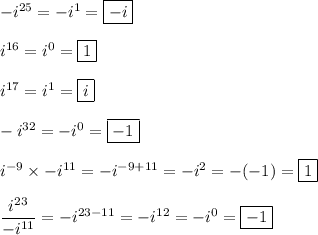Answer:
-i, 1, i, -1, 1, -1
Explanation:
You want the simplified form of various expressions involving powers of i.
Powers of i
The definition of i is ...
i = √(-1) or i² = -1
This value can be used to find other powers:
i³ = (i²)(i) = -i
i⁴ = (i²)² = (-1)² = 1
This means any power of i is equivalent to that power modulo 4.
Application