Question

This is a calculus question please make sure that all steps are shown and that everything is shown clearly i am terrible at calculus so please help .

Thank you

Answer 1

a)
`\displaystyle{(dG)/(dV_(\text out))=(20)/(V_(\text out)\ln 10)}`

b)
`\displaystyle{\frac{d^2G}{dV_\text{out}^2} = -\frac{20}{V_\text{out}^2\ln 10}}`

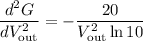
Explanation:

From the given equations to find, we are differentiating function G with respect to V out. Therefore, we will be only using the equation (6):

`\displaystyle{G=20\log (10V_(\text out))}`

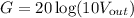
Here’s a fresh-up reminders for logarithmic differentiation formula:

`\displaystyle{f(V)=a\log_b V \to f'(x)=(a\cdot V')/(V\ln b)}`

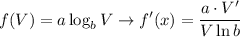
Note that V' means to derive or differentiate the V-term

Therefore, in this scenario, our values of term are:

• a = 20
• b = 10 (Since logarithm base is not shown, it’s always assumed to be common logarithm)
• V = 10V out

Therefore, we can apply differentiation formula:

`\displaystyle{(dG)/(dV_(\text out))=(20\cdot (d(10V_(\text out)))/(dV_(\text out)))/(10V_(\text out)\ln 10)}`

Simplify:

`\displaystyle{(dG)/(dV_(\text out))=(20\cdot 10)/(10V_(\text out)\ln 10)}\\\\\displaystyle{(dG)/(dV_(\text out))=(20)/(V_(\text out)\ln 10)}`

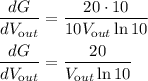
And we have finished the part a. On part b, we just differentiate the answer from part a again, the second part is to obtain the second derivative which is to derive the first derivative. Therefore:

`\displaystyle{(d^2G)/(dV_(\text out)^2) = (d\left((20)/(V_(\text out)\ln 10)\right))/(dV_(\text out))}`

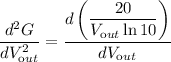
First, separate the constant every time when you have to differentiate a function:

`\displaystyle{(20)/(\ln 10)\cdot (1)/(V_(\text out))}`

Differentiate 1/V out: recall the formula of fraction:

`\displaystyle{f(V)=(1)/(V) =V^(-1) \to f'(x)=-V^(-2) = -(1)/(V^2)`

The formula above is derived from power rules formula where:

`\displaystyle{f(V)=V^n = nV^(n-1)}`

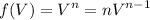
Therefore, from the function of V out:

`\displaystyle{\frac{d^2G}{dV_\text{out}^2} = (20)/(\ln 10) \cdot \left(\frac{1}{V_\text{out}}\right)'}\\\\\displaystyle{\frac{d^2G}{dV_\text{out}^2} = (20)/(\ln 10) \cdot (V_\text{out})^(-1)}\\\\\displaystyle{\frac{d^2G}{dV_\text{out}^2} = (20)/(\ln 10) \cdot (-V_\text{out})^(-2)}\\\\\displaystyle{\frac{d^2G}{dV_\text{out}^2} = (20)/(\ln 10) \cdot \left(-\frac{1}{V_\text{out}^2}\right)}`

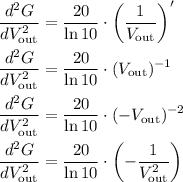
Simplify to latest as we get:

`\displaystyle{\frac{d^2G}{dV_\text{out}^2} = -\frac{20}{V_\text{out}^2\ln 10}}`

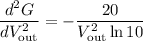
Please let me know if you have any questions!