Question

Which two lines are perpendicular to each other?

Line A: 2y=8x+10
Line B: 6y+3x = 12
Line C: 8y = −2x − 16

Answer 1

Line A and C

Explanation:

The perpendicular slope of another line is the negative reciprocal of the other slope.

For example, we have line
`x_(1)`.

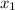y = x

The line that is perpendicular to it is:

y=
`-(1)/(x)`

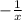
Lets tranform all of these equation to slope intercept form:

y = mx+b

M is the slope or how much the y value increases every time the x value increases by one. b is the y-intercept or when x=0.

Divide 2 on both sides to simplify line a into slope intercept form.

`(2y)/(2) = (8x+10)/(2)`

Simplify.

y = 4x + 5

Now it is in slope-intercept form. Slope of 4 and y-intercept of 5.

Line B

Isolate y on both sides by subtracting 3x on both sides.

6y + 3x - 3x = 12 - 3x

6y = 12 - 3x

Divide 6 on both sides.

`(6y)/(6) = (-3x+12)/(6)`

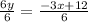
Simplify.

y = -1/2x + 2

Now it is in slope-intercept form. Slope of -1/2 and y-intercept of 2.

Line C

Divide both sides by 8.

`(8y)/(8) = (-2x-16)/(8)`

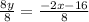
Simplify.

y = -1/4x - 2

Line A has a slope of 4. Line C has a slope of -1/4x. Line C is the negative reciprocal of Line A as multiplying Line A by -1 and flipping the fraction simple gets you Line C. (and vice versa).