Answer:
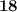
Step by step explanation:
![\: \: \: \: \: \: \: \bold{√(9 + 27) + 2 {}^(3) + \sqrt[3]{64} }](https://img.qammunity.org/2023/formulas/mathematics/high-school/281dzog6byhkrx028lwqau5kcbwmhq4rst.png)
To solve this problem, the first thing we have to do is simplify 3² to 9, since it multiplies 2 times 3 to give us 9.
![\: \: \: \: \: \: \: \: \: \: \: \: \bold{ √(36) + {2}^(3) + \sqrt[3]{64} }](https://img.qammunity.org/2023/formulas/mathematics/high-school/db01tsgcixcgs9r0e1bk2or4ng6rx2d8o6.png)
Then we must add 9 + 27 to give us a result of 36.
![\: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \bold{6 + {2}^(3) + \sqrt[3]{64} }](https://img.qammunity.org/2023/formulas/mathematics/high-school/s9o634wsf1kaw2qqlt9xlocz5mgddra6xd.png)
Now we must multiply again but this time we are going to multiply 2 times 6 since 6 × 6 is equal to 36.
![\: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \bold{6 + 8 \: + \sqrt[3]{64} }](https://img.qammunity.org/2023/formulas/mathematics/high-school/ykmntico5y9f96vr4d4gx6y891zl2ua3yd.png)
Now we must again multiply 3 times 2 to give us 8.
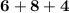
Then we must add 6 + 8 + 4.
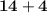
Before finishing we know that we got 14, now we must add 14 + 4 to give us a result of 18.
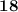
Rpt: The result is 18.