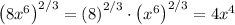The first step in simplifying is to apply that outer exponent of
 to each factor inside the parentheses.
to each factor inside the parentheses.

Now using the concept that
![(a)^(m/n) = \sqrt[m]{a^n}](https://img.qammunity.org/2023/formulas/mathematics/high-school/lkvz70iew6h2oses7ksmghtunr2mb2p1du.png) for positive real numbers a and integers m and n, we can evaluate the first part:
for positive real numbers a and integers m and n, we can evaluate the first part:
![(8)^(2/3) = \sqrt[3]{8^2} = \sqrt[3]{64} =4](https://img.qammunity.org/2023/formulas/mathematics/high-school/d6kucdq3h6pnakfmb08we7w53zcx70g5yb.png)
For the second part, we use the power-to-a-power rule, that says
 :
:

Putting that all together, we have