Question

Solve the equation
`(1)/(x+9) +(1)/(5)=(1)/(4)`

Answer 1

`\boldsymbol{\sf{Your\:exersice \to (1)/(x+9)+(1)/(5)=(1)/(4) }}`

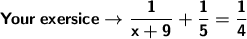
Variable x cannot be equal to −9 as division by zero is undefined. Multiply both sides of the equation by 20(x+9), the lowest common denominator of x+9,5,4.

`\boldsymbol{\sf{20+20(x+9)*\left((1)/(5)\right)=5(x+9) }}`

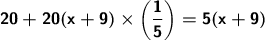
Multiply 5 and 1/5 to get 4.

`\boldsymbol{\sf{20+4(x+9)=5(x+9)}}`

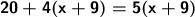
Use the distributive property to multiply 4 by x+9.

`\boldsymbol{\sf{20+4x+36=5(x+9)}}`

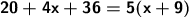
Add 20 and 36 to get 56.

`\boldsymbol{\sf{56+4x=5(x+9)}}`

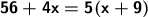
Use the distributive property to multiply 5 by x+9.

`\boldsymbol{\sf{56+4x=5x+45}}`

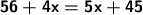
Subtract 5x on both sides.

`\boldsymbol{\sf{56+4x-5x=45}}`

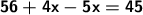
Combine 4x and −5x to get −x.

`\boldsymbol{\sf{56-x=45}}`

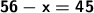
Subtract 56 from both sides.

`\boldsymbol{\sf{-x=45-56}}`

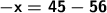
Subtract 56 from 45 to get −11.

`\boldsymbol{\sf{-x=-11}}`

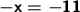
Multiply both sides by −1.

`\boldsymbol{\sf{x=11}}`