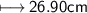2 Answers

Mikus · Answer 1 · 2023-11-06T09:17:07+0000

Hello..!

We use the Pythagorean formula:

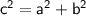
$\sf \red{c {}^(2) } = \blue{ a {}^(2) + b {}^(2) }$

We know that once we have the formula we extract the Raised data:

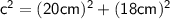
$\sf \red{c {}^(2)} = \blue{(20cm) {}^(2) + (18cm) {}^(2) }$

Having the Raised data, we will add as a power and we know that:

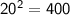
$\sf \red{20 {}^(2) } = \orange{400}$

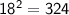
$\sf \red{18 {}^(2)} = \orange{324}$

So, to solve a power we must multiply squared and then get our result, in this case I added and then increased it by 0

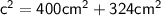
$\sf \red{c {}^(2) }= \blue{400cm {}^(2) + 324cm {}^(2) }$

We have the results, once that is done we will add.

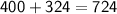
$\sf \red{400 + 324 }= \blue{724}$

So, we have the result we just have to put it like this:

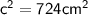
$\sf\red{c {}^(2) } = \blue{724cm {}^(2) }$

Once we have our result, we must take the square root, then the (square "²") automatically disappears.

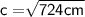
$\sf \red{c =} \green{ √(724cm) }$

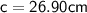
$\sf \red{c }= \orange{ 26.90cm}$

So, our result of our problem is:
$\orange{ \sf \longmapsto} \sf \blue{ \: 26.90cm}$

Please log in or register to add a comment.