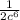Answer:
a.
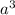 .
.
b.
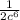 .
.
Explanation:
Exercise "a".
1. Write the expression.
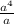
2. Subtract 1 from the exponent of both numerator and denominator.
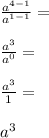
3. Final answer.
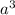
----------------------------------------------------------------------------------------------------------
Exercise "b".
1. Write the expression.
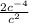
2. Move the numerator down to the denominator by changing the sign of the exponent.
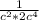
3. Multiply.