Question

One leg of a right triangle is 7 units long, and its hypotenuse is 16 units long. What is the length of the other leg? Round to the nearest whole number.

Answer 1

7^{2} + x^{2} = 16^{2}

49+x^2=256

x^2=207

x=14.387=14

Answer 2

14 units

Explanation:

To find the length of the other leg of a right triangle, where one leg is 7 units long and the hypotenuse is 16 units long, use Pythagoras Theorem.

Pythagoras Theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the legs.

Let the legs of the right triangle be "a" and "b", and the hypotenuse be "c". Therefore:

`\begin{aligned}a^2+b^2&=c^2\\a^2+7^2&=16^2\\a^2+49&=256\\a^2+49-49&=256-49\\a^2&=207\\√(a^2)&=√(207)\\a&=14.3874945...\\a&=14\;\sf(nearest\;whole\;number)\end{aligned}`

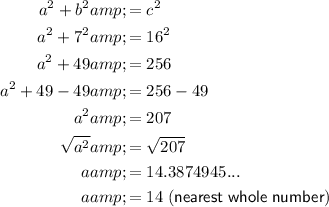
Therefore, the length of the other leg is 14 units (rounded to the nearest whole number).