Question

Let g(x)=log_2x1. find g(5)2. find g(-3)3. find g^-1(x)4. find g^-1(-3)

Answer 1

`g(x)=\log _2x`

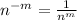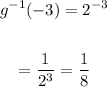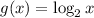
You evaluate the equation in the given values of x:

`\log _ba=(\log a)/(\log b)`

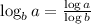
1. g(5)
`\begin{gathered} g(5)=\log _25 \\ g(5)=(\log 5)/(\log 2)=2.321 \end{gathered}`
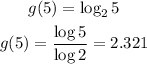
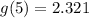
`g(5)=2.321`2. g(-3)

The logarithm of a negative number is undefined

3. g^-1(x)

To find the inverse function you:

-write the function with x and y:

`\begin{gathered} g(x)=\log _2x \\ y=\log _2x \end{gathered}`

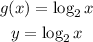
-Solve variable x:

knowing that:

`\begin{gathered} \log _ba=c \\ b^c=a \end{gathered}`
`\begin{gathered} y=\log _2x \\ \\ 2^y=x \end{gathered}`

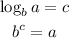
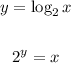
- Change the x for (g^-1(x)) and the y for x:

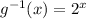
`g^(-1)(x)=2^x`4.g^-1(-3)​

As:

`n^(-m)=(1)/(n^m)`

`\begin{gathered} g^(-1)(-3)=2^(-3) \\ \\ =(1)/(2^3)=(1)/(8) \end{gathered}`