Given: Two functions as follows

Required: To find f(g(x)) and g(f(x)).
Explanation: f(g(x)) can be determined by putting g(x) in f(x) as follows
![f(g(x))=16[g(x)]^2](https://img.qammunity.org/2023/formulas/mathematics/college/ad97eq7c5e7f9w30d0ixa0kff65hlm6xmf.png)
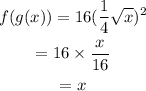
Similarly, for g(f(x)) we have
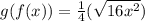
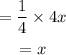
Since. f(g(x))=g(f(x)) the given functions f(x) and g(x) are inverse.
Final Answer: a) f(g(x)) is x
b) g(f(x)) is x
c) Functions f and g are inverse functions.