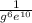ANSWER
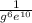
Step-by-step explanation
In the denominator, we have the product of g and g². The product of two powers with the same base is the base raised to the sum of the exponents,
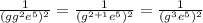
Now, we also have the power of a product. The exponents can be distributed into the multiplication,
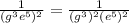
And finally, for both g and e, we have the power of a power. The result is the base raised to the product of the exponents,
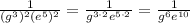
Hence, the simplified expression is,