There are 6 letters in the words GIGGLE, to be able to find the number of ways to arrange the letters, we will be using the Permutation Formula:
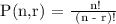
Where,
n = total number of objects in the set = number of letters in GIGGLE = 6
r = number of choosing objects from the set = be arranged in 6 letters still = 6
We get,
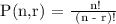
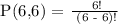

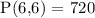
Therefore, there 720 ways to arrange the letters in GIGGLE.
The answer is 720.