Step-by-step explanation
Let the length be l and width b W, Since the length of the rectangle is 3 m less than twice the width, we will have
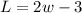
Therefore, the area becomes
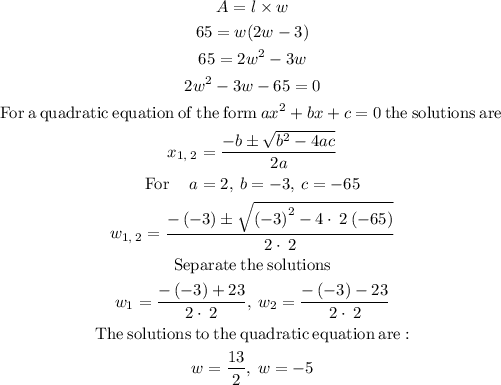
Logically, the width becomes
Answer: width = 6.5m
Therefore, the length becomes
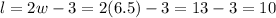
Answer" lenght =10 m