Question

Find the equation of a parallel to 5x-y=-3 that contains the points (-3,2) white the equation in slope intercept from

Answer 1

The given equation of a line is,

`5x-y=-3`

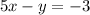
The above equation can be rewritten as,

`y=5x+3\text{ -----(1)}`

The general equation of a line in slope-intercept form is given by,

`y=mx+c\text{ ------(2)}`

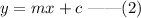
Here, m is the slope of the line and c is the y intercept.

Comparing equations (1) and (2), we get slope m=5.

We have to find the equation of a line parallel to the given line 5x-y=-3 and passing through point (x1, y1)=(-3,2).

The slopes of two parallel lines are always equal. Hence, the slope of a line parallel to 5x-y=-3 is m=5.

Now, the point-slope form of a line with slope m=5 and passing through point (x1, y1)=(-3,2) can be written as,

`\begin{gathered} m=(y1-y)/(x1-x) \\ 5=(2-y)/(-3-x) \end{gathered}`

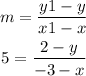
Rearrange the above equation in slope intercept form.

`\begin{gathered} 5(-3-x)=2-y \\ 5*(-3)-5x=2-y \\ -15-5x=2-y \\ y=5x+2+15 \\ y=5x+17 \end{gathered}`

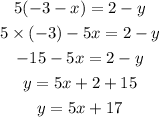
Therefore, the equation of a line parallel to the given line 5x-y=-3 and passing through point (-3,2) is y=5x+17.