Question

12. (ST5) The average length of illness for flupatients in a season is normally distributed,with a mean of 8 days and a standarddeviation of 0.9 day. What percentage of flupatients will be ill for more than 10 days?A. 1.31%B. 98.7%C. 5.62%D. 3.31%

Answer 1

Since the number of days is normally distributed, we can use a z-score table to find the percentage under a certain value in the distribution.

To do this, we first need to convert the value to a z-score by using:

`z=(x-\mu)/(\sigma)`

Where z is the z-score, x is the value we want to convert, μ is the mean and σ is the standard deviation.

Since we want the bound value of 10 days, we will convert this to z-score:

`z=(10-8)/(0.9)=(2)/(0.9)\approx2.222`

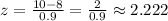
So, in a z-score table, we can consult the probability of having a value under a certain z-score. for this case, we have:

`P(z<2.222)\approx0.9869`

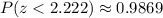
However, we want the probability, or the percentage, of patiens to get ill more than 10 days, in terms of z, this means:

`P(z>2.222)=?`

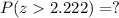
Since the whole normal distribution has a percentage of 100%, that is, 1, if we add the percentage less than and greater than 2.222, we wil have 1, so:

`\begin{gathered} P(z<2.222)+P(z>2.222)=1 \\ P(z>2.222)=1-P(z<2.222) \\ P(z>2.222)=1-0.9869 \\ P(z>2.222)=0.0131=1.31\% \end{gathered}`

Which corresponds to alternative A.