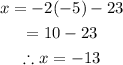Answer:
x = -13 and y = -5
Step-by-step explanation:
Given the below equations;

Let's go ahead and solve simultaneously using the substitution method.
From the 2nd equation;
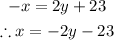
Let's put x = -2y - 23 into the 1st equation and find y;
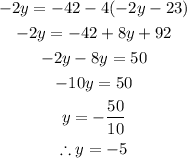
Let's substitute the value of y into x = -2y - 23 and find x;