The parabola function is given by,
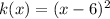
We need to find two points on the parabola (other than vertex and x-intercepts).
Let's take a point x = 1 and find the corresponding y value:
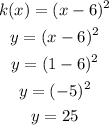
So, the point is (1, 25)
Now, let's take another random x value of x = 2,
So, the corresponding y value is:
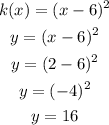
The other point is (2, 16)
Answer(s)