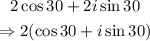ANSWER
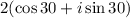
Step-by-step explanation
We want to convert the complex number to polar form:
![\sqrt[]{3}+i](https://img.qammunity.org/2023/formulas/mathematics/college/hqnfre6o1n9ajylv57j9qozgokmof2aeca.png)
The general polar form of a complex number is:
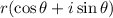
where:
![\begin{gathered} r=\sqrt[]{x^2+y^2} \\ \theta=\tan ^(-1)((y)/(x)) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/56nh9ymnwv1nnr2ckqb67cwzdn90wtk978.png)
Note: x is the real part of the complex number while y is the coefficient of i.
Therefore, from the number given:
![\begin{gathered} x=\sqrt[]{3} \\ y=1 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/cd0jqt96wmvalprrvvtrnsjufi3b8vz47b.png)
We now have to find r and θ:
![\begin{gathered} \Rightarrow r=\sqrt[]{(\sqrt[]{3})^2+1^2}=\sqrt[]{3+1} \\ r=\sqrt[]{4} \\ r=2 \\ \Rightarrow\theta=\tan ^(-1)(\frac{1}{\sqrt[]{3}}) \\ \theta=30\degree \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/8namu5uvmi6kjb9kvgdltxi3pqvws7xc47.png)
Therefore, the polar form of the complex number is: