To calculate the confidence interval, we have the formula:
![CI=\bar{x}\pm z(\frac{s}{\sqrt[]{n}})](https://img.qammunity.org/2023/formulas/mathematics/college/llm56h6pv52o7p5szmda57tgtz40rsaitn.png)
where bar x = mean, z = z-value, s = standard deviation, and n = sample size.
For a 99% confidence interval, the z-value is 2.58. Let's plug in the given information in the question to the formula above.
![CI=3.8\pm2.58(\frac{18.7}{\sqrt[]{44}})](https://img.qammunity.org/2023/formulas/mathematics/college/k8accwn3ptngbeqc81r64umqtwfsh42bjv.png)
Then, solve. Let's start by getting the quotient of 18.7 and square root of 44.
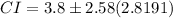
Next, multiply 2.58 and 2.8191.

Lastly, separate the plus and minus sign and do the operation.
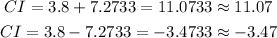
Hence, at 99% confidence interval, the population mean falls between -3.47 mg/dL and 11.07 mg/dL.
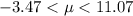
Since our confidence interval includes zero, this means that at some point if experiment is to be rerun again, there's a chance that the garlic treatment did not affect the LDL cholesterol levels. (Option C)