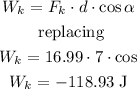Question

A mass M=15kg is pulled along a horizontal floor, with a coefficient of kinetic friction MK=0.15, for a distance of 7m. Then the mass is continued to be pulled up a frictionless incline that makes an angle theta=38 degrees with the horizontal. The entire time the massless rope used to pull the block is pulled parallel to the incline at an angle of theta=38 degrees (Thus on the incline it is parallel to the surface) and has a tension T=55N.1) What is the work done by tension before the block gets to the incline?2) What is the work done by friction as the block slides on the flat horizontal surface?

Answer 1

1) 303.38 J

2) -118.93 J

Explanation:

Given:

m = 15 kg

k = 0.15

d = 7 m

T = 55

Angle = 38°

1)

Work done by tension force is given as:

`\begin{gathered} W_t=T\cdot d\cdot\cos \theta \\ \text{ replacing:} \\ W_t=55\cdot7\cdot\cos 38 \\ W_t=303.38\text{ J} \end{gathered}`

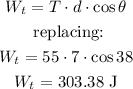
2)

For the mass , force equation in vertical direction is given as:

`\begin{gathered} T\cdot\sin \theta+F_n=m\cdot g \\ \text{ replacing} \\ 55\cdot\sin 38+F_n=15\cdot9.81 \\ F_n=113.29\text{ N} \end{gathered}`

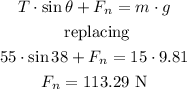
Kinetic frictional force is given as

`\begin{gathered} F_k=k\cdot F_n \\ F_k=0.15\cdot113.29 \\ F_k=16.99\text{ N} \end{gathered}`

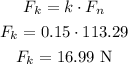
Work done by the frictional force is given as:

`\begin{gathered} W_k=F_k\cdot d\cdot\cos \alpha \\ \text{ replacing} \\ W_k=16.99\cdot7\cdot\cos _{} \\ W_k=-118.93\text{ J} \end{gathered}`