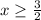Question

Solve the inequality 4x - 7 >= (-12x + 14) / 4

Answer 1

As given by the question:

There are given that the inequality:

`4x-7\ge(-12x+14)/(4)`

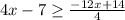
Now,

Multiply both sides by 4:

`\begin{gathered} 4x-7\ge(-12x+14)/(4) \\ (4x-7)*4\ge(-12x+14)/(4)*4 \\ (4x-7)*4\ge-12x+14 \\ 16x-28\ge-12x+14 \end{gathered}`

Then,

Add 28 to both sides in the above equation:

`\begin{gathered} 16x-28\ge-12x+14 \\ 16x-28+28\ge-12x+14+28 \\ 16x\ge-12x+42 \end{gathered}`

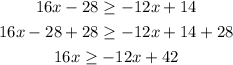
Then,

Add 12x to both sides in the above equation:

`\begin{gathered} 16x\ge-12x+42 \\ 16x+12x\ge-12x+42+12x \\ 28x\ge42 \end{gathered}`

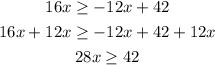
Then,

Divide both sides by 28:

`\begin{gathered} 28x\ge42 \\ (28x)/(28)\ge(42)/(28) \\ x\ge(3)/(2) \end{gathered}`

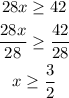
Hence, the value of the given inequality is shown below:

`x\ge(3)/(2)`