Question

For the reaction 2Al + 3CuCl₂ -> 3Cu + 2AlCl3, 20g of Al reacted with 90g of CuCl₂. The limiting reactant is (blank)because it produced the (blank) amount of copper

Answer 1

1. The first thing we do is chech if the reaction is balanced:

`2Al\text{ + 3}CuCl_2\rightarrow3Cu+2AlCl_3`

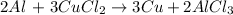
As we can see the equation is balanced because there are on both sides:

2 atoms of Al

3 atoms of Cu

6 atoms of Cl

2. Now we calculate the moles of each reactant that we have:

For this we need to know the molar mass of each reactant. We calculate it using the periodic table.

`\begin{gathered} M_(Al)=26.982\text{ }(g)/(mol) \\ M_(CuCl_2)=63.546\text{ }(g)/(mol)\text{ + }2\text{ }x\text{ }35.45(g)/(mol)=134.446(g)/(mol) \end{gathered}`

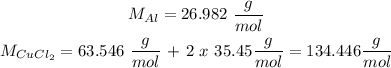
So we calculate:

`\begin{gathered} n_(Al)=(20g)/(26.982(g)/(mol))=0.74\text{ mol} \\ n_(CuCl_2)=(90g)/(134.446(g)/(mol))=0.66mol \end{gathered}`

3. Now we calculate the limiting reactant:

If we consider that all the aluminum reacts, for 0.74 moles we would need:

`n_(CuCl_2)=0.669\text{ }mol\text{ }x\frac{3\text{ }molCuCl_2}{2Al\text{ }}=1.11molCuCl_2`

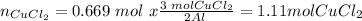
So we see that we need 1.11 moles of CuCl2 in order for the aluminum to react completely. So the limiting reactant is CuCl2.

Now we calculate the amount of copper produced:

So the amount of copper produced is 0.669 moles.