The correct area enclosed by the figure is 9538.56 m², not 1313.28 m² as claimed by Frank. Frank's error likely occurred when subtracting the area of the square from the area of the four semicircles.
Frank needs to determine the area enclosed by a figure created by attaching four semicircles to each side of a square with dimensions 48 m x 48 m.
1. Find the area enclosed by the figure.
2. Identify the error made by Frank, who initially claimed the area was 1313.28 m².
1. Find the area of the 4 semi-circles:
The area of one full circle is given by the formula A = πr², where r is the radius. Since these are semicircles, we need to halve the result.
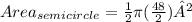
Calculate this for one semicircle, and then multiply by 4 for all four.
2. Find the area of the square:
Area_{square} = 48 × 48
3. The area enclosed by the figure:
Add the area of the 4 semicircles to the area of the square.
Area_{enclosed} = Area_{square} + Area_{4 semicircles}
Error made by Frank:
Frank claimed the area was 1313.28 m², but this seems to be incorrect.
Area_{enclosed} = 4 × 1/2 × π × (48/2)² + 48 × 48
Area_{enclosed} = 4 × 1/2 × 3.14 × 24² + 48 × 48
Area_{enclosed} = 4 × 3.14 × 576 + 48 × 48
Area_{enclosed} = 7234.56 + 2304
Area_{enclosed} = 9538.56 m²
Complete question:
Frank needs to check the area enclosed by the figure. The figure is made by attaching semi circles to each side of a 48 m x 48 m². Frank says the area is 1313. 28 m². find the area enclosed by the figure. use 3.14 for pie. what error might Frank have made?