Question

I need help question

Answer 1

Hello there. To solve this question, we'll have to remember some properties about derivatives.

Given the following function:

`f(x)=(5\tan(x))/(x)`

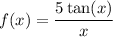
We want to take its derivative.

For this, we'll have to apply some rules:

The derivative of a quotient of two continuously differentiable functions is given by the quotient rule:

`\left((f(x))/(g(x))\right)'=(f'(x)\cdot\,g(x)-f(x)\cdot\,g'(x))/((g(x))^2)`

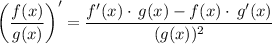
The derivative of the tangent function can be found using the above rule and by knowing the derivatives of sine and cosine. In this case, we obtain the following:

`\begin{gathered} (\tan(x))^(\prime)=\left((\sin(x))/(\cos(x))\right)^(\prime)=((\sin(x))'\cdot\cos(x)-\sin(x)\cdot(\cos(x))')/((\cos(x))^2) \\ \\ \Rightarrow(\cos^2(x)+\sin^2(x))/(\cos^2(x))=(1)/(\cos^2(x))=\sec^2(x) \end{gathered}`

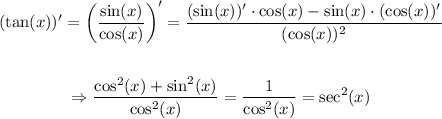
The derivative of a power can be found using the limit definition of a derivative, in this case we obtain:

`(x^n)^(\prime)=n\cdot\,x^(n-1),\text{ }n\in\mathbb{R}`

With these rules, we can already solve the question.

First, the derivative is a linear operator, which means that

`(\alpha\cdot\,f(x))^(\prime)=\alpha\cdot\,f^(\prime)(x),\text{ }\alpha\in\mathbb{R}`

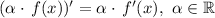
Hence we get that

`f^(\prime)(x)=\left((5\tan(x))/(x)\right)^(\prime)=5\cdot\left((\tan(x))/(x)\right)^(\prime)`

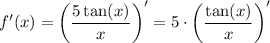
Now, apply the quotient rule

`f^(\prime)(x)=5\cdot((\tan(x))'\cdot\,x-\tan(x)\cdot(x)')/(x^2)`

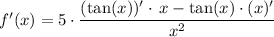
Take the derivative of the tangent and apply the power rule

`f^(\prime)(x)=5\cdot(\sec^2(x)\cdot\,x-\tan(x)\cdot1)/(x^2)`

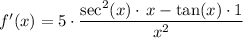
Therefore we get that

`f^(\prime)(x)=5\cdot(x\sec^2(x)-\tan(x))/(x^2)`

Is the answer to this question.