Question

You wish to take a flight from Nevada to New York. Use the point (-4,0) to represent Nevada, aand the (5,2) to represent New York. The airline had partitioned the flight so that you have a layover. The flight distance is partitioned in a ratio 3:2. Determine the ordered pair that represents the location of the layover. What state will you be in when you have the layover?

Answer 1

To answer this question we will use the following formulas to compute the coordinates of the point that divides a segment with terminal points (x₁,y₁) and (x₂,y₂) at a given ratio a:b:

`\begin{gathered} x=(bx_1+ax_2)/(b+a), \\ y=\frac{by_1_{}+ay_2}{b+a}\text{.} \end{gathered}`

Therefore, the coordinates that represent the location of the layover are:

`\begin{gathered} x=(2*(-4)+3*5)/(2+3), \\ y=(2*0+3*2)/(2+3)\text{.} \end{gathered}`

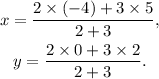
Simplifying the above results we get:

`\begin{gathered} x=(-8+15)/(5)=(7)/(5)=1.4, \\ y=(0+6)/(5)=(6)/(5)=1.2. \end{gathered}`

Now, from the given map, we get that the point (1.4,1.2) is on Iowa.

Answer:

The ordered pair that represents the location of the layover is:

`\mleft(1.4,1.2\mright)\text{.}`

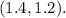
The state at which we will be during the layover is Iowa.