Question

15)Identify a possible first step using the elimination method to solve the system and then find the solution to the system.3x - 5y = -22x + y = 3A)Multiply Rt equation by -3 and second equation by 2, solution (-1, 1).EliminateB)Multiply first equation by -2 and second equation by 3, solution (1, 1).CMultiply first equation by -3 and second equation by 2, solution (1, -1).D)Multiply first equation by -2 and second equation by 3, solution (1, -1).

Answer 1

`\text{ B\rparen Multiply first equation by -2 and second equation by 3, solution \lparen1,1\rparen}`

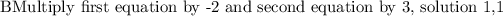
Explanation:

Solve the following system of equations using the elimination method.

The elimination method is the process of eliminating one of the variables in the system of linear equations using the addition or subtraction methods in conjunction with the multiplication or division of coefficients of the variables.

Therefore,

`\begin{gathered} 3x-5y=-2\text{ * \lparen-2\rparen = -6x+10y=4} \\ 2x+y=3\text{ * \lparen3\rparen = 6x+3y=9} \end{gathered}`

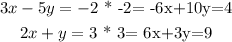
Now, compute and solve for ''y'':

`\begin{gathered} 13y=13 \\ y=(13)/(13) \\ y=1 \\ \end{gathered}`

Then, plug the ''y'' value into one of the functions:

`\begin{gathered} x=(3)/(2)-(y)/(2) \\ x=(3)/(2)-(1)/(2) \\ x=(2)/(2)=1 \end{gathered}`

Hence, the solution of the system is (1,1).