Question

A rectangular coordinate system with coordinates in miles is placed with the origin at the center of a city. The figure to the right indicates that a university is located 3.7 miles west and 3.9 miles south of the center of the city. A seismograph on the campus shows that a small earthquake occurred. The quake's epicenter is estimated to be approximately 32 miles from the university. Write the standard form of the equation for the set of points that could be the epicenter of the quake.

Answer 1

The standard circle equation is

`(x-x_0)^2+(y-y_0)^2=r^2`

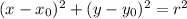
Where r is the radius of the circle, and O = (x₀, y₀) is the center of the circle.

In our problem, the radius is 32, and the center of the circle is (-3.7, -3.9), then we have

`\begin{gathered} r=32 \\ x_0=-3.7 \\ y_0=-3.9 \end{gathered}`

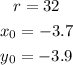
Now we just have to put these values on our circle equation

`\begin{gathered} (x-x_0)^2+(y-y_0)^2=r^2 \\ \\ (x-(-3.7))^2+(y-(3.9)_{}_{})^2=32^2 \\ \\ (x+3.7)^2+(y+3.9_{})^2=1024 \end{gathered}`

The standard form of the equation is

`(x+3.7)^2+(y+3.9_{})^2=1024`