Question

Find the measure of angle B.a=63.63 mi, b= 43.59 mi, C= 45.4 degrees

Answer 1

Let us begin by illustrating the problem using a diagram:

To find the measure of angle B, we need to first find the length of the side opposite angle C using cosine rule.

Cosine rule is defined to be:

`c^2\text{ = a}^2\text{ + b}^2\text{ - 2abCosC}`

Substituting the given sides and angle:

Let c be the side opposite angle C, b be the side opposite angle B and a be the side opposite angle A

`\begin{gathered} c^2\text{ = 63.63}^2\text{ + 43.59}^2\text{ - 2}*\text{ 63.63 }*\text{ 43.59 }*\text{ cos45.4} \\ c^2\text{ = 2053.837} \\ c\text{ =}√(2053.837) \\ c\text{ = 45.32 mi} \end{gathered}`

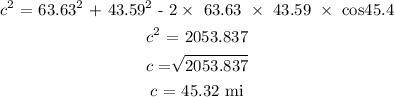
Hence, we have the triangle:

The next step is to use sine rule to find the measure of angle B

sine rule is defined as:

`\frac{sin\text{ A}}{a}=\frac{sin\text{ B}}{b}\text{ = }\frac{sin\text{ C}}{c}`

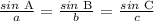
Applying sine rule:

`\begin{gathered} \frac{sin\text{ C}}{c}=\text{ }\frac{sin\text{ B}}{b} \\ \frac{sin\text{ 45,4}}{45.32\text{ }}\text{ = }\frac{sin\text{ B}}{43.59} \\ sin\text{ B= }\frac{sin45.4\text{ }*\text{ 43.49}}{45.32} \\ sin\text{ B = 0.68327} \\ B=\text{ }\sin^(-1)0.68327 \\ B\text{ = 43.0997} \\ B\text{ }\approx\text{ 43.1}^0 \end{gathered}`

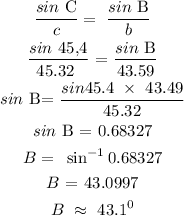
Answer:

Measure of angle B = 43.1 degrees