We have on the denominator the next expression:

The function is not defined when the denominator is equal to 0.
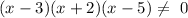
So the function is undefined when
x-3 =0
x+2=0 and
x-5 = 0
Solving for x:
x=3
x=-2
x=5.
With the before information, we can find that Bobby is right, the function is undefined when x=3. x=-2 and x=5.