The transformation given tells us to add 2 to the x-coordinate (the first in the ordered pair x,y) and subtract 2 from the y-coordinate (the second in the ordered pair x,y).
That is what (x, y) -> (x + 2, y - 2) means.
Then, we can now easily work on each of the 4 coordinates given:
For C:
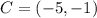
The x-coordinate is -5 and the y coordinate is -1. As stated at the beginning, we need to add 2 to the x-coordinate to form the new x-coordinate (the one of C'). That will be -5 + 2 = -3.
Subtracting 2 from the y-coordinate as instructed for the y-coordinate of C', we have -1 - 2 = -3.
Then:
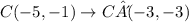
For the other coordinates we just need to follow the same logic:
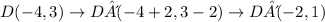
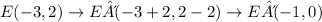
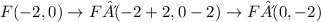
Then, the blanks should be filled as follows:
C'(-3, -3)
D'(-2, 1)
E'(-1, 0)
F'(0, -2)